A polygon has multiple sides. The number of sides depends on the shape of the polygon.
Polygons are closed two-dimensional shapes with straight sides. They can have any number of sides, but they must have at least three sides. A polygon with three sides is called a triangle, a polygon with four sides is called a quadrilateral, a polygon with five sides is called a pentagon, and so on.
The sum of the interior angles of a polygon is always (n-2) x 180 degrees, where n is the number of sides. Polygons are commonly found in geometry, architecture, art, and nature. Understanding the properties of polygons is essential in mathematics and various other fields.
The Polygon Spectrum
Welcome to the fascinating world of polygons! In this blog post, we will explore the concept of the polygon spectrum, which encompasses the wide range of shapes that polygons can take. From triangles to n-gons, the possibilities are infinite. Let’s dive in and discover the beauty and diversity of polygons!
From Triangles To N-gons
At the beginning of the polygon spectrum, we have the simplest and most fundamental polygon: the triangle. With its three sides and three angles, the triangle serves as the building block for all other polygons. It is the starting point for exploring the diverse range of shapes that lie ahead.
As we move along the spectrum, we encounter polygons with more sides, such as the quadrilateral (four sides), pentagon (five sides), hexagon (six sides), and heptagon (seven sides). Each polygon has its own unique characteristics and properties, making them intriguing subjects of study in geometry.
But the spectrum doesn’t stop there. It extends infinitely, allowing us to explore polygons with an increasing number of sides, known as n-gons. An n-gon is a polygon with “n” sides, where “n” can be any positive integer. This means that there is no upper limit to the number of sides a polygon can have!
The Infinite Possibilities
The polygon spectrum opens up a world of infinite possibilities. With each additional side, polygons become more complex and intricate. They can exhibit symmetrical patterns, fascinating angles, and captivating symmetry. From regular polygons with equal side lengths and angles to irregular polygons with varying side lengths and angles, the spectrum offers a vast array of shapes to explore.
Not only do polygons have aesthetic appeal, but they also have practical applications in various fields. Architects use polygons to design buildings with unique and visually appealing facades. Artists incorporate polygons into their artwork to create geometric compositions. Engineers utilize polygons in structural designs to ensure stability and efficiency.
Understanding the polygon spectrum allows us to appreciate the beauty and versatility of these shapes. Whether you’re a geometry enthusiast, an artist, or simply curious about the world around you, exploring the polygon spectrum can be an exciting and enlightening journey.

Credit: www.flickr.com
Sides And Shapes
In the world of geometry, polygons are fascinating shapes that come in various forms and sizes. Understanding the relationship between the sides and shapes of polygons can provide valuable insights into their properties and characteristics.
The Simplest Polygons
Basic polygons are the building blocks of geometric shapes. They are defined by their straight sides and enclosed spaces, commonly referred to as vertices. The simplest polygons are known as triangles and quadrilaterals. These polygons have a set number of sides, with triangles having three sides and quadrilaterals having four sides.
Complex Figures Explained
As we move beyond the basic polygons, we encounter more complex figures with a greater number of sides. These shapes include pentagons (5 sides), hexagons (6 sides), heptagons (7 sides), octagons (8 sides), and so on. Each of these polygons exhibits unique properties and can be found in various natural and man-made objects, showcasing the diversity and versatility of polygonal shapes.
Mathematical Foundations
Understanding the mathematical foundations of polygons is essential to grasp the concept of how many sides a polygon can have. In this section, we will explore the geometric definitions of polygons and the crucial role that angles play in determining the number of sides.
Geometric Definitions
Before delving into the number of sides a polygon can have, let’s begin by understanding what a polygon is. A polygon is a closed two-dimensional shape with straight sides, formed by connecting multiple line segments. These line segments, known as edges, intersect at points called vertices. Each vertex is where two adjacent sides of the polygon meet.
Furthermore, polygons can be classified based on the number of sides they possess. For example, a polygon with three sides is called a triangle, while a polygon with four sides is known as a quadrilateral. As the number of sides increases, polygons can be referred to as pentagons (five sides), hexagons (six sides), heptagons (seven sides), and so on.
The Role Of Angles
Angles play a crucial role in determining the properties of polygons, including the number of sides they can have. An angle is formed by two intersecting line segments, and it measures the amount of rotation between these segments. In the case of polygons, the sum of all interior angles depends on the number of sides.
For instance, in a triangle, the sum of all interior angles is always 180 degrees. As the number of sides increases, the sum of interior angles also increases. In a quadrilateral, the sum of interior angles is 360 degrees, while in a pentagon, it is 540 degrees. The pattern continues, with each additional side contributing an additional 180 degrees to the total sum of interior angles.
By understanding the relationship between angles and the number of sides, we can determine the maximum and minimum number of sides a polygon can have. The minimum number of sides is three (a triangle), while there is no maximum limit to the number of sides a polygon can possess.
To summarize, the mathematical foundations of polygons involve understanding their geometric definitions and the relationship between angles and the number of sides. This knowledge allows us to explore the vast world of polygons and appreciate their diverse forms and properties.
Classifying Polygons
Regular Vs. Irregular
A regular polygon has equal sides and equal angles. An irregular polygon has unequal sides and angles.
Convex Vs. Concave
A convex polygon has all its interior angles less than 180 degrees and all line segments connecting any two points within the polygon lie entirely inside the polygon. A concave polygon has at least one interior angle greater than 180 degrees and may have line segments that lie partially outside the polygon.
The Significance Of Sides
A polygon is a closed figure in a plane with straight sides. One of the most fundamental attributes of a polygon is its sides. The number of sides a polygon has determines its name and impacts its properties. In this section, we will explore the significance of sides in polygons.
Determining The Polygon’s Name
The number of sides in a polygon directly determines its name. Let’s take a look at some common polygons and their names based on the number of sides:
| Number of Sides | Polygon Name |
|---|---|
| 3 | Triangle |
| 4 | Quadrilateral |
| 5 | Pentagon |
| 6 | Hexagon |
| 7 | Heptagon |
| 8 | Octagon |
Side Count Impact On Properties
The number of sides in a polygon affects its properties, including its angles and diagonals. Here are some key points to consider:
- A triangle, with three sides, has interior angles that always add up to 180 degrees.
- A quadrilateral, with four sides, can have a variety of shapes and angles.
- A regular polygon, with equal side lengths and angles, has a special property where the interior angles are determined by the formula (n-2) 180 degrees, where n represents the number of sides.
- As the number of sides increases, the sum of the interior angles also increases.
- The number of diagonals in a polygon can be calculated using the formula n (n-3) / 2, where n is the number of sides.
Understanding the significance of sides in a polygon helps us classify and analyze different shapes. By knowing the number of sides, we can determine the polygon’s name and gain insights into its properties. Whether you’re a student studying geometry or simply curious about the world of shapes, the number of sides in a polygon plays a vital role in understanding its characteristics.
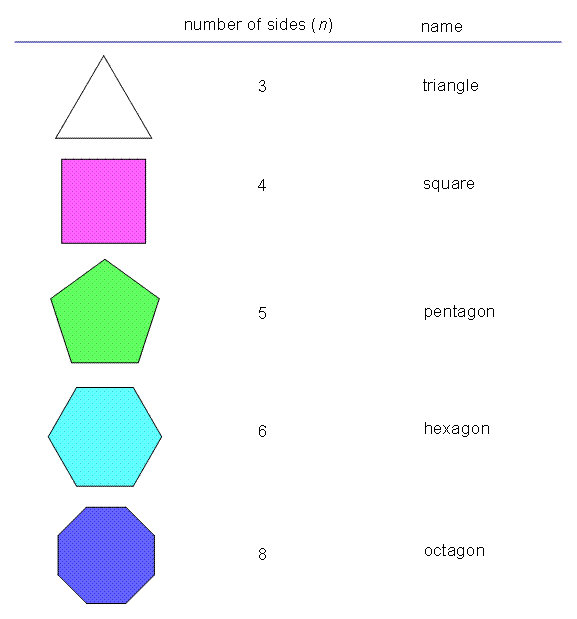
Credit: americanboard.org
Polygon In The Real World
A polygon is a closed figure made up of straight lines, and it is a fundamental geometric shape that we encounter in our everyday lives. Let’s explore how polygons manifest in the real world, from architectural marvels to nature’s mesmerizing patterns.
Architectural Marvels
Architectural marvels such as the Pyramids of Giza in Egypt and the Pantheon in Rome showcase the timeless beauty of polygons in their construction. The pyramids, with their triangular sides, stand as a testament to the significance of polygons in architecture.
Nature’s Geometric Patterns
Nature’s geometric patterns often exhibit the symmetry and elegance of polygons. From the hexagonal shapes of honeycombs to the pentagonal designs of snowflakes, polygons are prevalent in the intricate formations found in nature.
Calculating Perimeters And Areas
Discovering the number of sides a polygon has is crucial when calculating perimeters and areas. By counting the sides, you can accurately determine the shape’s dimensions for precise mathematical calculations.
Perimeter Formulas
Calculating the boundary length around a polygon is crucial. Here are common perimeter formulas:
- Triangle: Sum of all three sides
- Rectangle: 2 x (length + width)
- Square: 4 x side length
- Regular Polygon: Number of sides x side length
Area Calculation Methods
Determining the space inside a polygon involves various methods:
- Triangle: 0.5 x base x height
- Rectangle: Length x width
- Square: Side length squared
- Regular Polygon: 0.5 x perimeter x apothem
Advanced Polygon Studies
Discover the world of Advanced Polygon Studies by exploring the intriguing question: How many sides does a polygon have? Delve into the fascinating realm of geometric shapes and uncover the secrets behind the varying number of sides in different polygons.
Polygons In Higher Dimensions
Exploring polygons in dimensions beyond 2D opens up a world of mathematical possibilities.
Applications In Computer Graphics
Utilizing polygons in computer graphics enhances visual realism and object representations.
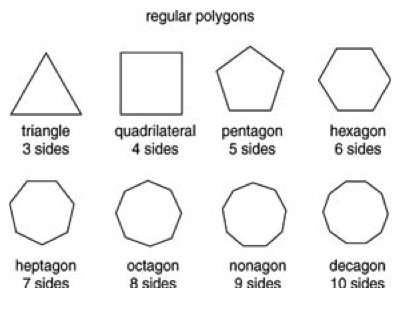
Credit: socratic.org
Frequently Asked Questions
How Many Sides Does A Polygon Have?
A polygon is a two-dimensional shape with straight sides. It can have any number of sides, from 3 to infinity. Polygons with 3, 4, 5, 6, 7, 8, 9, 10, 11, and 12 sides are commonly known as triangles, quadrilaterals, pentagons, hexagons, heptagons, octagons, nonagons, decagons, hendecagons, and dodecagons, respectively.
What Is The Difference Between A Polygon And A Regular Polygon?
A polygon is any two-dimensional shape with straight sides, while a regular polygon is a polygon with all sides and angles congruent (equal). All regular polygons are also polygons, but not all polygons are regular.
Can A Polygon Have Curves Instead Of Straight Sides?
No, by definition, a polygon is a two-dimensional shape with straight sides. If a shape has curves, it is called a curved shape or a curved polygon.
What Is The Formula To Calculate The Sum Of Interior Angles In A Polygon?
The formula to calculate the sum of interior angles in a polygon is (n-2) x 180 degrees, where n is the number of sides in the polygon. For example, a hexagon has 6 sides, so the sum of its interior angles is (6-2) x 180 = 720 degrees.
Conclusion
Understanding the number of sides in a polygon is crucial for geometry enthusiasts. Whether it’s a triangle, quadrilateral, or any other shape, the sides define its structure. By grasping this concept, you can appreciate the beauty and complexity of polygons in various mathematical and real-world scenarios.
Explore and enjoy geometry!


